tyrandoupup 2019-05-02 10:19 采纳率: 0%
浏览 4358
已采纳

neo4j 节点如何选择在graph中显示那个属性

如图，我已经导入数据。但是graph图中不显示内容，如何选择自己想显示的节点属性？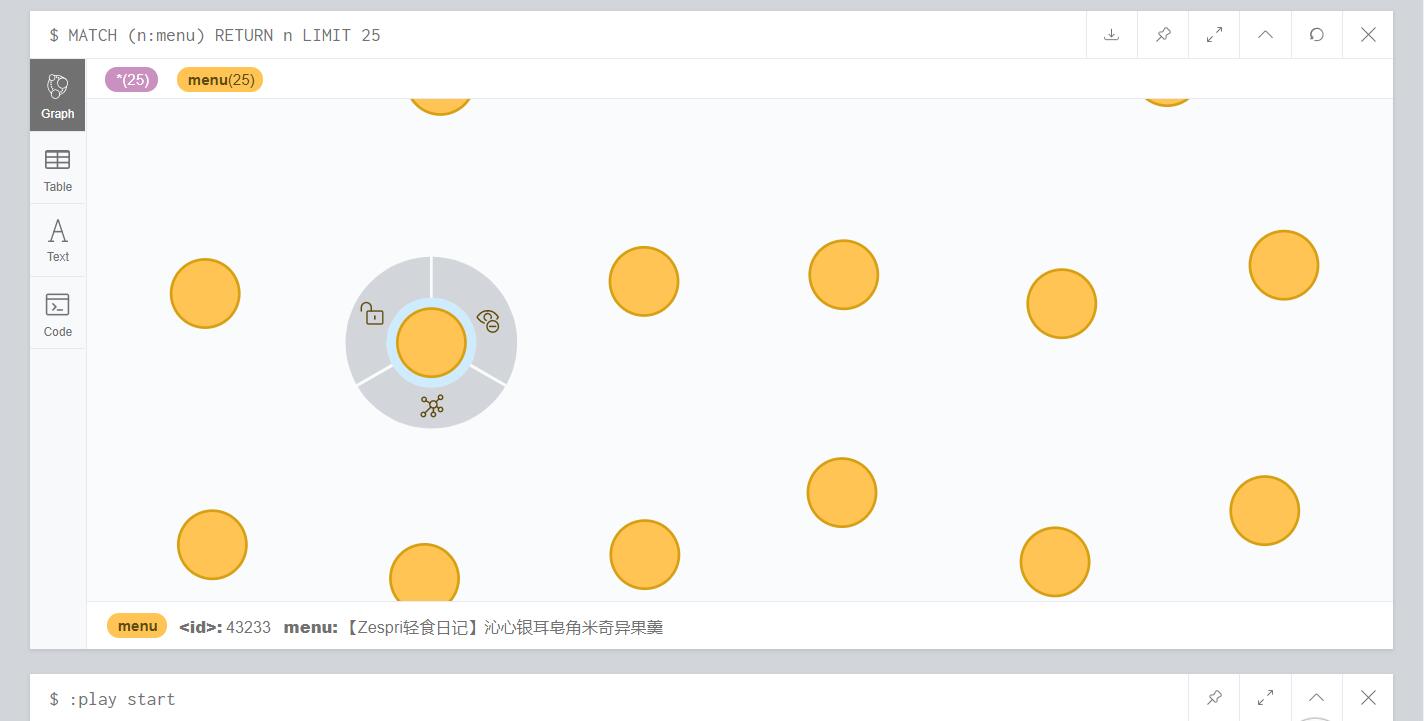


图片说明

  • 写回答

2条回答 默认 最新

  • tyrandoupup 2019-05-08 21:56
    关注

    已解决，点击图中menu(25)，然后在下面选择即可

    本回答被题主选为最佳回答 , 对您是否有帮助呢?
    评论
查看更多回答(1条)

报告相同问题？

悬赏问题

  • ¥15 在若依框架下实现人脸识别
  • ¥15 网络科学导论，网络控制
  • ¥100 安卓tv程序连接SQLSERVER2008问题
  • ¥15 利用Sentinel-2和Landsat8做一个水库的长时序NDVI的对比，为什么Snetinel-2计算的结果最小值特别小，而Lansat8就很平均
  • ¥15 metadata提取的PDF元数据，如何转换为一个Excel
  • ¥15 关于arduino编程toCharArray()函数的使用
  • ¥100 vc++混合CEF采用CLR方式编译报错
  • ¥15 coze 的插件输入飞书多维表格 app_token 后一直显示错误，如何解决？
  • ¥15 vite+vue3+plyr播放本地public文件夹下视频无法加载
  • ¥15 c#逐行读取txt文本，但是每一行里面数据之间空格数量不同