用Python动画证明采样定理（奈奎斯特定理）

Question

用Python编写程序，证明采样定理（香农采样定律、奈奎斯特采样定律）
请用动画描述这一定理。分为两种情况f s≥2f max和f s<2f max。
请对下边代码进行完善，使其符合要求，有动画效果

import numpy as np
import matplotlib.pyplot as plt

# 生成信号
Fmax = 20
t = np.linspace(0, 1, 1000)
signal = np.sin(2 * np.pi * Fmax * t)

# 采样
Fs1 = 20
Ts1 = 1 / Fs1
samples1 = np.sin(2 * np.pi * Fmax * np.arange(0, 1, Ts1))
Fs2 = 60
Ts2 = 1 / Fs2
samples2 = np.sin(2 * np.pi * Fmax * np.arange(0, 1, Ts2))
# 重建信号
reconstructed1 = np.zeros_like(t)
for i, s in enumerate(samples1):
    reconstructed1 += s * np.sinc(Fs1 * (t - i * Ts1))
reconstructed2 = np.zeros_like(t)
for i, s in enumerate(samples2):
    reconstructed2 += s * np.sinc(Fs2 * (t - i * Ts2))
# 绘图
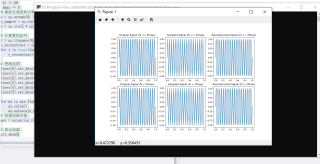
fig, axs = plt.subplots(3, 2, figsize=(8, 6), sharex=True, sharey=False)
axs[0,0].plot(t, signal)
axs[0,0].set_title('Original Signal 1 ')
axs[1,0].stem(np.arange(0, 1, Ts1), samples1, use_line_collection=True)
axs[1,0].set_title('Samples 1 ')
axs[2,0].plot(t, reconstructed1)
axs[2,0].set_title('Reconstructed Signal 1 ')
axs[0,1].plot(t, signal)
axs[0,1].set_title('Original Signal 2 ')
axs[1,1].stem(np.arange(0, 1, Ts2), samples2, use_line_collection=True)
axs[1,1].set_title('Samples 2 ')
axs[2,1].plot(t, reconstructed2)
axs[2,1].set_title('Reconstructed Signal 2 ')
plt.tight_layout()
plt.show()
 

Answer 1

运行结果如下：

代码如下：

import numpy as np
import matplotlib.pyplot as plt
import matplotlib.animation as animation

#设置采样频率
fs = 80

#设置信号最高频率
fmax = 20

#生成连续信号
x = np.linspace(0, 1, 1000)
y = np.sin(2 * np.pi * fmax * x)

fig, axs = plt.subplots(nrows=2, ncols=3, figsize=(12, 8))

#初始化子图
axs[0, 0].set_title('Original Signal (fs >= 2fmax)')
axs[0, 1].set_title('Sampled Signal (fs >= 2fmax)')
axs[0, 2].set_title('Reconstructed Signal (fs >= 2fmax)')
axs[1, 0].set_title('Original Signal (fs < 2fmax)')
axs[1, 1].set_title('Sampled Signal (fs < 2fmax)')
axs[1, 2].set_title('Reconstructed Signal (fs < 2fmax)')

lines = []
for ax in axs.flatten():
    line, = ax.plot([], [])
    lines.append(line)

#设置动画参数
frames = np.arange(1, 6)
interval = 2000
repeat_delay = 5000

def update(frame):

#设置新的采样频率和最高频率
 global fs, fmax
 fs += 20
 fmax += 5
# 重新生成采样点和连续信号
n = np.arange(0, 1, 1 / fs)
y_sample = np.sin(2 * np.pi * fmax * n)
y = np.sin(2 * np.pi * fmax * x)

# 计算重构信号
t = np.linspace(0, 1, len(x))
y_reconstruct = np.zeros_like(t)
for i in range(len(n)):
    y_reconstruct += y_sample[i] * np.sinc(fs * (t - n[i]))

# 更新绘图
lines[0].set_data(x, y)
lines[1].set_data(n, y_sample)
lines[2].set_data(x, y_reconstruct)
lines[3].set_data(x, y)
lines[4].set_data(n, y_sample)
lines[5].set_data(x, y_reconstruct)

for ax in axs.flatten():
    ax.relim()
    ax.autoscale_view()
# 创建动画对象
ani = animation.FuncAnimation(fig, update, frames=frames, interval=interval, repeat_delay=repeat_delay)

# 显示动画
plt.show()